Duration and Convexity explained
- Riot Investment Society
- Mar 21, 2024
- 7 min read
WHAT IS DURATION?
Duration is a measure used in bond markets to calculate the efficiency of a bond or portfolio and to measure their sensitivity to interest rate changes. It is also used as a measure of the volatility of a bond; therefore, as the duration increases, the volatility increases and therefore the risk of the bond. Duration is expressed in days or years and represents the time needed to return from the initial investment, that is, the time needed for the bond to repay with coupons and the final return on the capital invested initially.
It is important not to confuse duration with maturity, which indicates the maturity of a bond, or the date on which the lender is obliged to return the nominal value of the bond to the owner. However, maturity cannot be considered a reliable measure of the term life of a bond because it does not consider the time distribution of cash flows. Duration and maturity, in fact, coincide only in the case of Zero-Coupon Bonds as they provide only one cash flow at the end of the contract.
Duration was formulated in 1938 by Canadian economist Frederick Robertson Macaulay, from whom it takes the name of "Macaulay Duration". Mathematically, the indicator represents the average maturity of the cash f lows of a bond, weighted by the present value of the flows.
Where n is the number of maturity periods, 𝐹𝐶𝑘 is the cash flow to the k-th period, P is the current price of the bond, 𝑡𝑘 is the time between the coupon and the currency date expressed in years and r is the yield to maturity.
Let’s assume for example that you have a BTP with a maturity of 15/09/22, annual coupon of 1.45% with half- yearly accrual on dates 15/03 and 15/09 and that the currency date is 29/04/20. We also assume that the bond price is 101.70 and the yield to maturity 0.80%. The duration calculation is done as follows:
Finally, the sum of all discounted and multiplied 𝑡𝑘 cash flows is calculated, which amounts to 238,513, and is then divided by the bond price, resulting in a duration of 2,345.
ELEMENTS AFFECTING THE DURATION CALCULATION
By analysing the duration formula, it is possible to identify the elements that influence it, causing an increase or a reduction:
Maturity of the bond: it is the most important element in the duration calculation. An increase in the maturity of the bond increases the duration of the bond, resulting in a higher risk, as it is more sensitive to price changes.
Coupon: an increase in the coupon rate involves a reduction in duration, as the time needed to return from the investment is reduced. The coupon therefore has an inverse effect on duration.
Bond price: it also has an inverse effect on duration. An increase in price, in fact, involves a reduction in duration, while a reduction in price implies an increase.
Interest rate: by influencing the price of the bond, the interest rate has the same effect on duration. An increase in the interest rate will reduce the duration.
MODIFIED DURATION
The price sensitivity of a bond is calculated using the modified duration, which represents the percentage change in the price of a bond based on the percentage change in yield to maturity. The modified duration is calculated as the ratio of duration to the current rate of return of the bond (1+yield to maturity):
A very volatile bond is exposed to strong fluctuations in value in the face of changes in rates of return; therefore, the less volatility and duration of a bond the more attractive it is.
Graphically the duration represents the first derivative of the curve of the relation price-yield of a fixed income bond; therefore, use the duration means to move on the tangent straight line to the curve that represents the relation price yield.
A negative relationship can be seen in focusing on the price-yield curve, which means that a reduction in the interest rate increases the price of the bond more than an increase in the interest rate of an equal measure reduces the price of the bond. The modified duration is a precise estimate in case of contained rate changes while in case of greater variations the error committed by duration is amplified. The modified duration is also an approximation that tends to underestimate the rise in the price of the bond because of a reduction in the interest rate and to overestimate the negative change in price at increases in the interest rate.
IMMUNIZATION THEOREM
The immunization theorem is often used as part of the management strategies of bond portfolios and represents a technique according to which the purchase of a security with duration equal to the holding period allows to obtain a return equal to yield to maturity. This is because the change in the market price of a bond because of a change in rates is offset by the higher or lower yield resulting from the re-use of periodic cash flows.
The theorem becomes useful especially when applied to bond portfolios as it is possible to diversify the maturities, while for individual securities it becomes more complex as hardly a trader invests only in bond with duration exactly equal to the desired investment time horizon.
USE OF DURATION
It is important to note that under the bond portfolios, duration is calculated as the average duration of the securities that compose it. In particular, the use of duration is of fundamental importance in the management of portfolios to adapt them to changes in rates. It becomes indeed opportune to reduce the duration in the case of forecast increase of the rates, to reduce the volatility. However, reducing the duration reduces the risk and therefore the return. On the contrary, when a reduction of the rates is previewed, it is better to increase the duration.
VARIABLE RATE AND INFLATION-LINKED BONDS DURATION
Modified duration and duration are more accurate on fixed rate bonds and zero-coupon bonds than on variable rate and inflation-linked bonds.
Variable rate bonds, or bonds with an interest rate indexed to market rates, would have zero duration if the indexation was daily; Thus, their market price would not be affected by changes in interest rates because the coupon would adapt to the new market conditions. However, coupons do not ripen daily, so they do not adapt day by day to changes in market rates but ripen at intervals (usually half-yearly) and are fixed in advance on the day of maturing. This means that for these bonds’ duration is not zero but it’s lower than that of fixed coupon bonds. These bonds are therefore less risky.
Inflation-linked bonds have a duration that can be calculated as the duration of fixed rate bonds but using real prices. Inflation-linked bond volatility is linked to changes in the real interest rate, which is much less volatile than the nominal rate. For this reason, the duration of inflation-linked bonds at the same residual maturity is lower than that of fixed-rate bonds.
CONVEXITY
What is Convexity? We just defined duration as the percentage change in a bond price in response to a 1% change in yields, thus expressing a linear function. If this is true, how can we justify the curvature in the graphs representing this relationship?
The curves are sloped and not linear, and that’s because duration does not accurately express the relationship between bond’s price and yields, since it is an approximation, and it is not valid for large yields variation. To give a more accurate estimate, we must add another measure next to duration: the Convexity.
Indeed, convexity expresses the curvature of the curve, and a greater curvature corresponds to a higher convexity. In another term, a bond’s convexity measures the sensitivity of a bond’s duration to changes in yield. Convexity is calculated by the following formula:
According to the Taylor Series Expansion, a more accurate approximation of the price change of a bond following a change in interest rates can now be obtained by the following formula:
Now, let’s substitute the values of duration (D) and convexity (C):
N.B: the convexity of a bond portfolio tends to be higher when payments are evenly distributed over a long period of time. It is instead minimal when payments are concentrated around a particular date.
If both the net duration and convexity of a bond portfolio are equal to zero, the portfolio holder is immunized against parallel shifts, both small and large, of the yield curve, but remains exposed to non-parallel shifts.
Positive and Negative Convexity
There are two types of bond convexity: positive and negative convexity. A bond has positive convexity if its duration rises as the yield declines. A bond with positive convexity will have larger price increases due to a decline in yields than price declines due to an increase in yields. Positive convexity can be thought of as working in the investor’s favor, since the price becomes less sensitive when yields rise, and so prices go down, than when yields decline, and so prices go up. Bonds can also have negative convexity, which would indicate that duration rises as yields increase and can work against an investor’s interest.
Below are listed typical examples of bonds with positive convexity and negative convexity:
Positive Convexity: Non-callable bonds, bonds with make-whole calls.
Negative Convexity: MBS (Mortgage-Backed Securities), bonds with a traditional call, preferred.
A useful way to visualize a bond’s convexity is to plot the potential price change against various yields. If two bonds have the same duration and yield but differing convexities, a change in interest rates will affect each bond differently. For example, the chart below shows three bonds: a bond with higher positive convexity (Bond A) will be less affected by interest rates than a bond with lower positive convexity (Bond B). On the other hand, a bond with negative convexity (Bond C) will exhibit larger price fluctuations should rates rise than if they were to fall.
In conclusion, Duration and Convexity are two metrics used to help investors understand how the price of a bond will be affected by changes in interest rates. How a bond’s price responds to a change in interest rates is measured by its duration and can help investors understand the implications for a bond’s price should interest rates change. The change in a bond’s duration for a given change in yields can be measured by its convexity.
If rates are expected to increase, an investor should consider bonds with shorter durations. These bonds will be less sensitive to a rise in yields and will fall in price less than bonds with higher durations.
If rates are expected to decline, an investor should consider bonds with higher durations. As yields decline and bond prices move up, higher duration bonds stand to gain more than their lower duration counterparts.


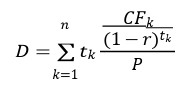








Comments